In Section 7.4 we analyzed the impulse response of a recirculating comb filter, of which the one-pole low-pass filter is a special case. Figure 8.22 shows the result for two low-pass filters and one complex one-pole resonant filter. All are elementary recirculating filters as introduced in Section 8.2.3. Each is normalized to have unit maximum gain.
In the case of a low-pass filter, the impulse response gets longer (and
lower) as the pole gets closer to one. Suppose the pole is at a point ![]() (so that the cutoff frequency is
(so that the cutoff frequency is ![]() radians). The normalizing factor is
also
radians). The normalizing factor is
also ![]() . After
. After ![]() points, the output diminishes by a factor of
points, the output diminishes by a factor of
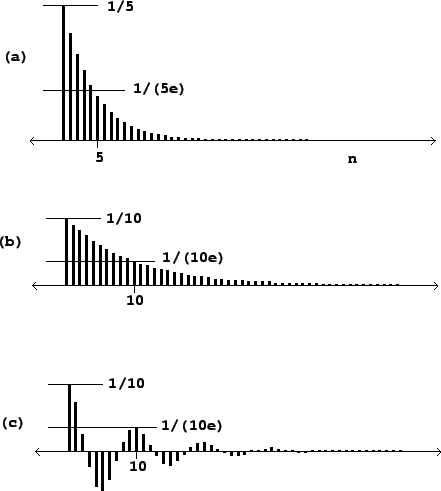 |
The situation gets more interesting when we look at a resonant one-pole filter,
that is, one whose pole lies off the real axis. In part (c) of the figure,
the pole ![]() has absolute value 0.9 (as in part b), but its argument is
set to
has absolute value 0.9 (as in part b), but its argument is
set to ![]() radians. We get the same settling time as in part (b), but
the output rings at the resonant frequency (and so at a period of 10 samples
in this example).
radians. We get the same settling time as in part (b), but
the output rings at the resonant frequency (and so at a period of 10 samples
in this example).
A natural question to ask is, how many periods of ringing do we get before the
filter decays to strength ![]() ? If the pole of a resonant filter has magnitude
? If the pole of a resonant filter has magnitude
![]() as above, we have seen in Section 8.2.3 that the
bandwidth (call it
as above, we have seen in Section 8.2.3 that the
bandwidth (call it ![]() ) is about
) is about ![]() , and we see here that the settling time
is about
, and we see here that the settling time
is about ![]() . The resonant frequency (call it
. The resonant frequency (call it ![]() ) is the argument of the
pole, and the period in samples of the ringing is
) is the argument of the
pole, and the period in samples of the ringing is
![]() . The number of periods that make up the settling time is thus:
. The number of periods that make up the settling time is thus: